
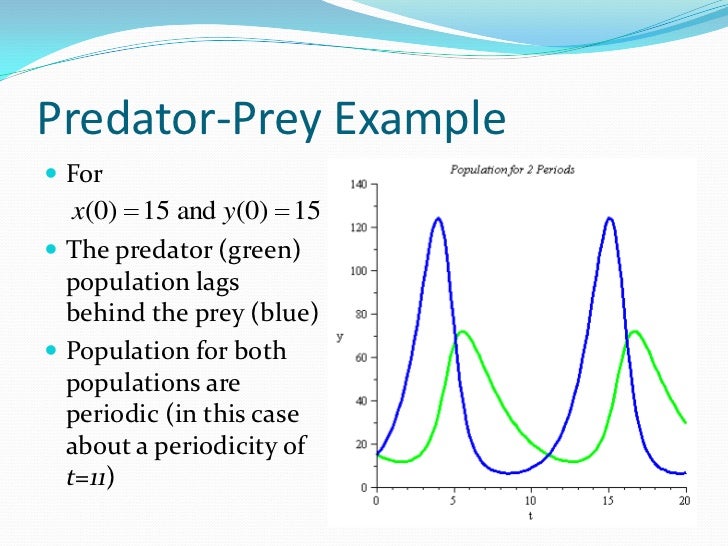
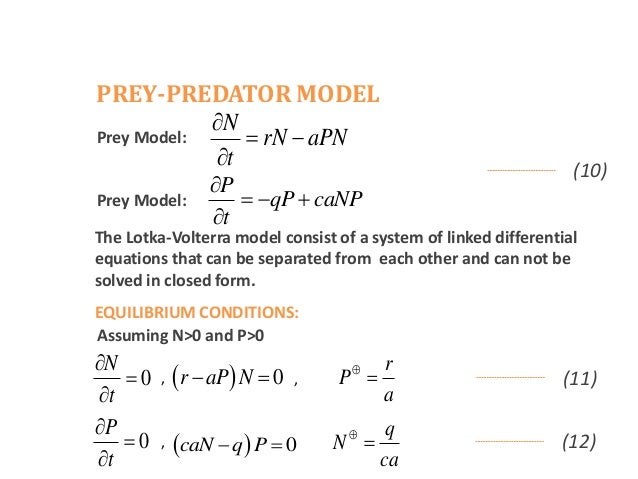
5 discussed the global dynamics of a predator-prey system with defense mechanism. 4 reveal the complex dynamical behavior of prey-predator system with group defense, Xu et al. 3 investigated the stability and global dynamic of a predator-prey model with group defense, Raw et al. To further grasp the law of interaction of predator species and prey species, many scholars pay much attention to the group defense mechanism of the prey. In the natural ecosystem, different biological populations take certain measures such as group defense, refuging, escaping and so on, to maintain their own survivals or search for food. Since the classical works of Lotka and Volterra 1, 2, the research on the dynamical behavior of predator-prey models has become a crucial research field for mathematicians and biologists. It is well known that the interaction of predator species and prey species is an important topic in biology and mathematical ecology. Finally, simulation results are presented to substantiate the theoretical findings. Thirdly, the existence of Hopf bifurcation of the considered predator-prey model is investigated by using the Hopf bifurcation theory of fractional order differential equations. Secondly, we analyze the existence of various equilibrium points by basic mathematical analysis method and obtain some sufficient criteria which guarantee the locally asymptotically stability of various equilibrium points of the involved predator-prey model with the aid of linearization approach. Firstly, we establish the sufficient conditions that guarantee the existence, uniqueness and boundness of solution by applying Lipschitz condition, inequality technique and fractional order differential equation theory. The dynamical properties such as the existence, uniqueness and boundness of solution, the stability of equilibrium point and the existence of Hopf bifurcation of the involved predator-prey model have been discussed. In the present article, a new fractional order predator-prey model with group defense is put up.


 0 kommentar(er)
0 kommentar(er)
